How can beamforming create power?

In this short article we describe a very interesting but mind-bugging phenomenon in beamforming. Mathematically, it seems that beamforming can create infinite power from a constant power.

Let us assume that there is a point source that generates a sound wave travelling outward spherically. The power source has a constant positive power of P. When it reaches to a microphone at position T with a power of Q. We know that the power is attenuated inversely proportional to the square of the distance. However that has no relevance to the problem.

Let us further assume that the sound wave is sinusoidal. Therefore, the amplitude will be proportionally to the squared root of the power. The amplitude of the sound wave at T will be

$A_T\propto\sqrt Q$

Figure 1: Transmitter Power P and Receiver Power Q

Now let us divide the constant power P evenly to two sources. As shown below, each source will reach the microphone with power Q/2. The amplitude from each will be

$A_i\propto\sqrt{Q/2}$

Figure 2: Splitting Power Evenly into Two Sources

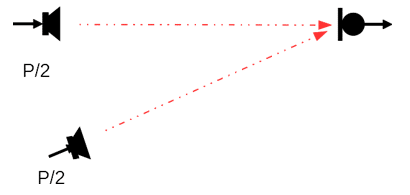
Assume that by some magic we align both the sinusoidal signals at the receiver in phase. The summed amplitude of the microphone signal will have an amplitude

$A_1+A_2\propto2\sqrt{Q/2}$

and in terms of power, 2Q. Therefore, we receive two times the power compared with without beamforming whilst the total transmission power stays the same.

Let us further generalize the idea to N transmitters. We divide the constant power P into N evenly distributed sound sources. Each source will have a power of P/N. At the receiver end each will contribute a power Q/N and a sinusoidal wave of amplitude $\sqrt{Q/2}$ . When perfect beamforming is applied to the N incoming signals, we have the combined single waveform with magnitude $N\sqrt{Q/N}$ . The total power received becomes NQ.

Now we have an issue. No matter how small Q is compared to P, we can always find an integer N such that the following holds,

$P\ <\ NQ$ .

Through beamforming, we actually receive more power than the total power transmitted! Where is the power coming from?

Beamforming is a great technique. However, there are fundamental principles it cannot violate.

Complete Communications Engineering