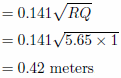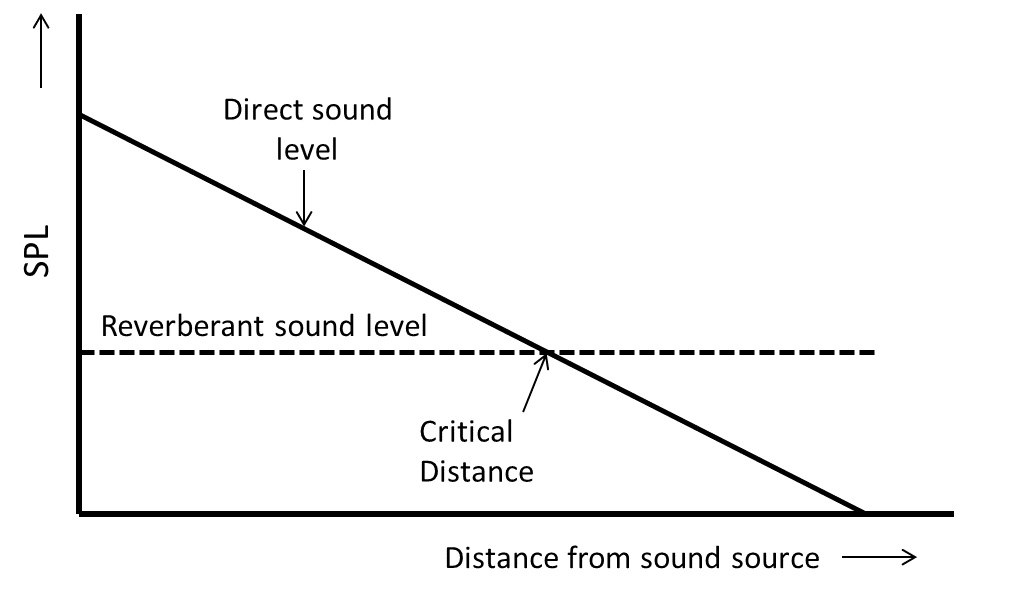
When designing a sound or a control room, the concept of critical distance is an important factor for positioning loudspeakers and microphones. Critical distance is the distance from the sound source at which the reverberant sound level and the direct signal level are equal. When standing at less than the critical distance a listener resides predominantly in the direct sound field. At any location beyond the critical distance a listener is in the reverberant field.
As an example, consider the case of a sound emitted from a loudspeaker into a room. After the initial sound transmission (and during), both a direct and reverberant sound field will develop in the surrounding area. At any given time then, the room will contain a combination of both the direct signal, and the reverberant sound field (which consists of reflected waves from boundaries). The direct sound field decays by 6dB for each doubling of distance from the sound source, while the reverberant sound level should remain reasonably uniform in all parts of the room.
Frequency Dependence
The critical distance is also frequency dependent. Lower frequencies tend to radiate omnidirectionally and hence hit more boundaries more frequently. In an average domestic room, the low frequency critical distance can be as short as half a meter. A general equation for Critical Distance (DC) is given by:
where R is the room constant, and Q is the directivity factor of the loudspeaker. The room constant (R) can be shown to be:
where S is the room surface area (m2), and A is the mean absorption coefficient of the room surfaces. The Q can be visualized as the portion of a sphere that is insonified by the directfield of a loudspeaker, with a value of 1 given by an omnidirectional loudspeaker radiating in freespace. For low-frequency radiation from a freestanding loudspeaker (which is typically omnidirectional), a Q of 1 is always a good approximation.
As a simple example, consider a room of surface area 50m2, with plasterboard walls and an average amount of furnishing. The absorption coefficient of such a room could likely be around 0.15. The room constant (R) could then be found as:
Consequently, the critical distance in the room for the low frequency radiation would be given by:
As expected, the critical distance is quite short. At higher frequencies, the directivity factor of a loudspeaker increases, which results in a corresponding increase in the critical distance.
When recording, microphones are often placed inside the direct sound field to achieve high speech intelligibility. At any significant distance outside of the critical distance a substantial amount of speech intelligibility can be lost. Many recording studios will place their microphones inside of the critical distance, and then add artificial reverb during subsequent mixdown. In this way, they can decide how much reverb must be added to give a realistic brightening to the recording.
References
[1] Newell, P., Recording Studio Design, 3rd ed., Focal Press, Burlington, MA, 2013, Chapter 11, pp. 339-341.
[2] Winer, E., The Audio Expert: Everything You Need to Know About Audio, Focal Press, Burlington, MA, 2013, Part1, pp. 304-306.